The points \(P\) and \(Q\) on the ellipse
\[\dfrac{x^2}{a^2}+\dfrac{y^2}{b^2}=1\]
have coordinates \((a\cos\theta, b\sin \theta)\) and \((-a\sin\theta, b\cos\theta)\) respectively. Show that, if \(O\) is the origin,
- \(OP^2+OQ^2 = a^2 + b^2\),
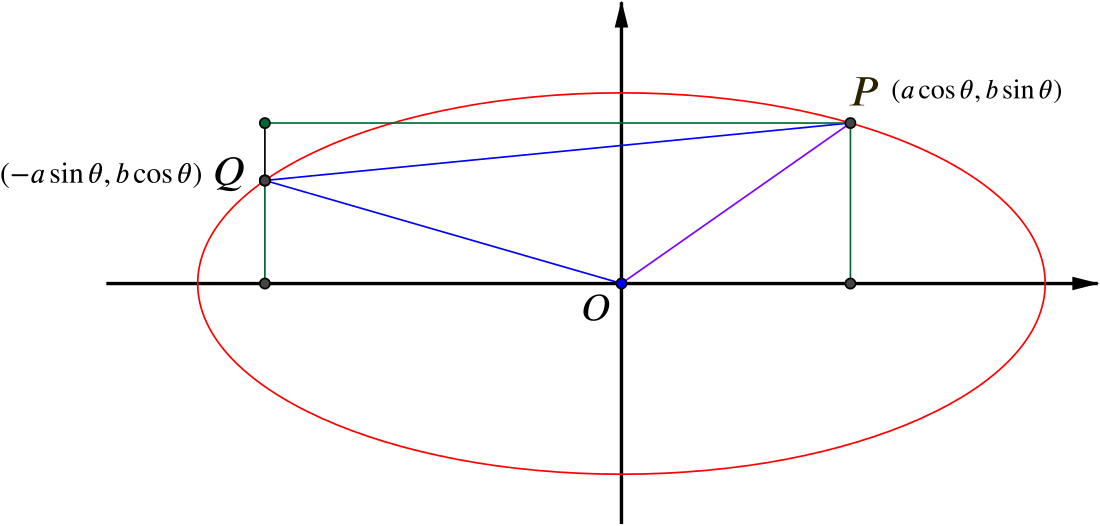
Let \(c = \cos \theta\) and \(s = \sin \theta\), and so \(c^2 + s^2 = 1.\)
Thus \(OP^2+OQ^2 = (ac-0)^2 + (bs-0)^2 + (-as-0)^2 + (bc-0)^2 =a^2(c^2+s^2) + b^2(s^2+c^2) = a^2 + b^2\).
- the area of triangle \(OPQ\) is \(\dfrac{1}{2}ab\),
The area of triangle \(OPQ\) is the area of the rectangle in the diagram with three right-angled triangles subtracted.
So the area of triangle \(OPQ\) is
\[(ac+as)(bs)-(0.5ac\times bs)-(0.5as\times bc) -0.5(ac+as)(bs-bc)\]
\[= abcs+abs^2-0.5abcs-0.5abcs -0.5abcs+0.5abcs -0.5abs^2 +0.5abc^2\]
\[= \dfrac{ab}{2}.\]
We have here chosen the case when \(b\sin \theta > b\cos\theta\), but this method will deal with all possible cases.
- the midpoint of \(PQ\) always lies on the curve whose equation is
\[\dfrac{2x^2}{a^2}+\dfrac{2y^2}{b^2}=1.\]
The midpoint of \(PQ\) is the point \((\dfrac{ac-as}{2}, \dfrac{bc+bs}{2}) =(x,y).\)
Thus \(\dfrac{2x}{a}= c-s, \dfrac{2y}{b} = c+s.\) Squaring each equation and then adding them gives
\[\dfrac{4x^2}{a^2}+\dfrac{4y^2}{b^2} =2,\]
and so the midpoint of \(PQ\) has the locus
\[\dfrac{2x^2}{a^2}+\dfrac{2y^2}{b^2}=1.\]
We can see the locus traced out by \(A\) in the GeoGebra applet below.

