Find the equation of the circle which passes through the points \((2,0)\) and \((8,0)\) and touches the \(y\)-axis on the positive side of the origin.
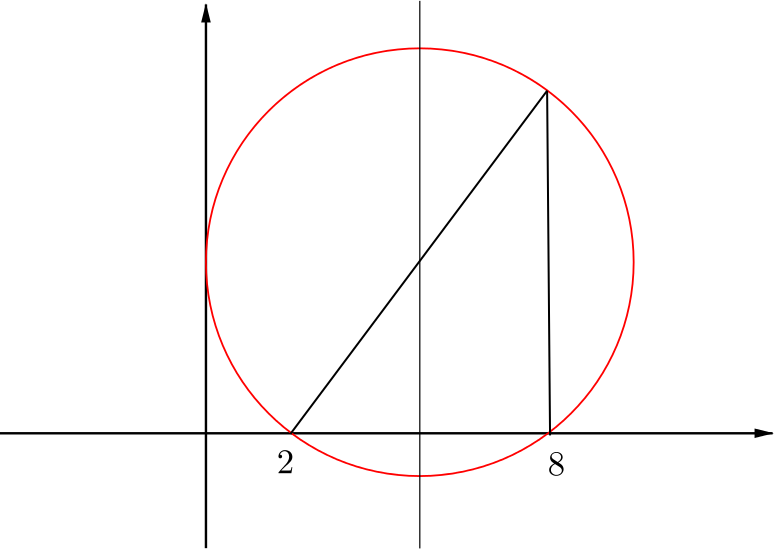
The vertical line of symmetry of the circle is \(x = 5\), and so the centre of the circle must be a point of the form \((5,q)\).
As the circle touches the \(y\)-axis, the radius of the circle is therefore \(5\).
The distance between \((2,0)\) and \((5,q)\) must be \(5\), so \(\sqrt{(2-5)^2 + q^2} = 5\). Solving for \(q\) gives \(q = 4\), and so the centre of the circle is \((5,4)\).
Therefore the equation of the circle is \[ (x - 5)^2 + (y - 4)^2 = 25. \]
Find the coordinates of the point on the circle which is furthest from the point \((2,0)\).
The point on the circle furthest from this point lies on the opposite end of the diameter that contains \((2,0)\).
By symmetry around the centre \((5,4)\), this point is \((8,8)\).

