Alice, Bob and Charlie are well-known expert logicians; they always tell the truth.
They are sat in a row, as illustrated above. In each of the scenarios below, their father puts a red or blue hat on each of their heads. Alice can see Bob’s and Charlie’s hats, but not her own; Bob can see only Charlie’s hat; Charlie can see none of the hats. All three of them are aware of this arrangement.
- Their father puts a hat on each of their heads and says: “Each of your hats is either red or blue. At least one of you has a red hat.” Alice then says “I know the colour of my hat.” What colour is each person’s hat? Explain your answer.
Alice only knows the colours of the other two hats, and that at least one hat is red.
From this she has deduced the colour of her own hat, so Bob and Charlie’s hats must be BB (both blue) - if Bob and Charlie are RR or RB or BR, this won’t help Alice enough to be sure of her own hat.
Therefore she must have deduced that she was wearing the one red hat.
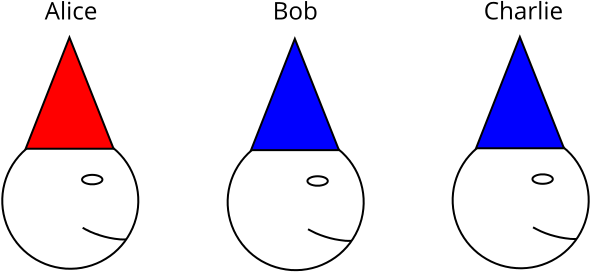
- Their father puts a new hat on each of their heads and again says: “Each of your hats is either red or blue. At least one of you has a red hat.” Alice then says “I don’t know the colour of my hat.” Bob then says “I don’t know the colour of my hat.” What colour is Charlie’s hat? Explain your answer.
Alice doesn’t know the colour of her hat, and so either Bob, Charlie or both has a red hat.
Bob, knowing this, and knowing the colour of Charlie’s hat, still doesn’t know the colour of his own hat.
This means that Charlie must have a red hat - if he has a blue one, Bob can deduce his own hat is red.
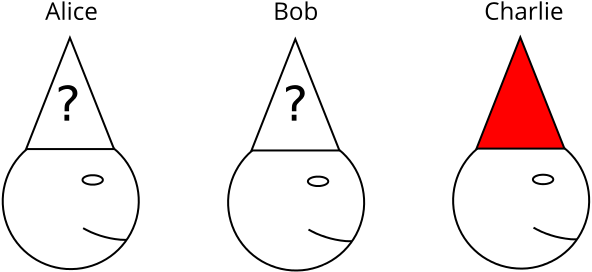
- Their father puts a new hat on each of their heads and says: “Each of your hats is either red or blue. At least one of you has a red hat, and at least one of you has a blue hat.” Alice says “I know the colour of my hat.” Bob then says “Mine is red.” What colour is each person’s hat? Explain your answer.
Alice knows the colours of Bob and Charlie’s hats, and that there’s at least one hat of each colour.
From this she has deduced the colour of her own hat, so Bob and Charlie must have the same colour hats, and Alice has the other colour.
Bob knows this, and knows the colour of Charlie’s hat, which must be red, allowing Bob to deduce his own hat is red.
Therefore Alice’s hat is blue, Bob’s is red, and Charlie’s is also red.

- Their father puts a new hat on each of their heads and says: " Each of your hats is either red or blue. At least one of you has a red hat, and at least one of you has a blue hat." Alice then says “I don’t know the colour of my hat.” Bob then says “My hat is red.” What colour is Charlie’s hat? Explain your answer.
Alice knows that there is at least one hat of each colour, and she can see the colours of Bob’s and Charlie’s hats.
She can’t deduce the colour of her own hat, so Bob and Charlie must have different coloured hats.
Bob knows this, and can see the colour of Charlie’s hat.
Therefore Charlie’s hat must be blue, allowing Bob to deduce that his own hat is red.
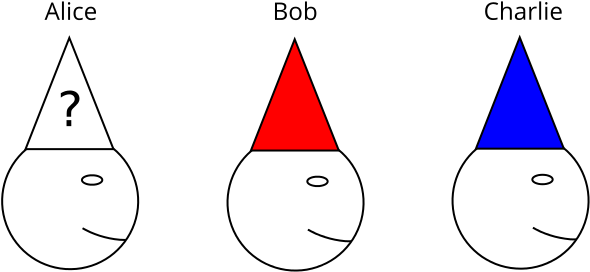
- Their father puts a new hat on each of their heads and says: “Each of your hats is either red or blue. Two of you who are seated adjacently both have red hats.” Alice then says “I don’t know the colour of my hat.” What colour is Charlie’s hat? Explain your answer.
The only possibilities are RRB, BRR, and RRR.
The first of these enables Alice to deduce she has a red hat, so this can’t happen.
Therefore Bob and Charlie must both have red hats.


