In the four-by-four version of the game, starting with pattern
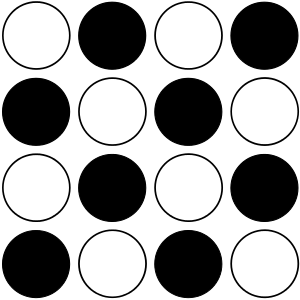
explain why it is impossible to reach a pattern with only one white counter.
When a row or a column is flipped, what happens to the number of whites in that row or column?
In the five-by-five game, explain why any sequence of moves which begins
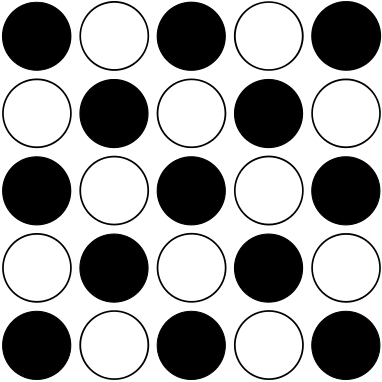
and ends with an all-white pattern, must involve an odd number of moves. What is the least number of moves needed? Give reasons for your answer.
Again, when a row or a column is flipped, what happens to the number of whites in that row or column?
Can we see how to get to an all-white board? Can we show that it can’t be done in fewer moves?

