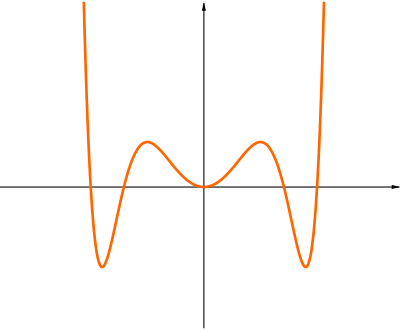
An even function is a function \(f(x)\) with the property that \(f(-x)=f(x)\) for any value of \(x\). The graph of \(y=f(x)\) for an even function has reflectional symmetry about the \(y\)-axis.
Examples are \(\cos x\) and \(x^2\).
Note that it is possible for a function to be neither even nor odd, for example \(f(x)=1+2x\).

