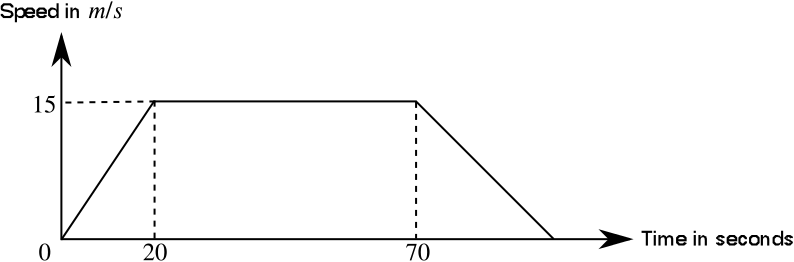
The diagram shows a speed-time graph of a car.
- Calculate the acceleration of the car during the first \(20\) seconds.
The acceleration at any point is given by the gradient of the line, so the acceleration is constant on each section.
The gradient for the first section is \(\dfrac{15}{20}=0.75\), and so the acceleration of the car during the first \(20\) seconds is \(\quantity{0.75} {m/s^2}\).
- Calculate the distance the car travels from rest before it begins to decelerate.
The distance the car travels is given by the area below the graph up to \(t = 70s\).
We’ll split this area into two pieces, the left hand side triangle (the distance the car travels during the first 20 seconds), and the rectangle for \(20s \leq t \leq 70s\).
The base of the triangle is \(20\) and the height is \(15\), and so the area of the triangle is given by \(\dfrac{20 \times 15}{2} = 150\).
The rectangle has sides of lengths \(15\) and \(70-20 = 50\) and so has an area of \(15 \times 50 = 750.\)
Therefore, the total distance the car travels before it begins to decelerate is \(150 + 750 = \quantity{900}{m}\).
- Given that the car decelerates at \(\quantity{0.5} {m/s^2}\), calculate the total time taken for the journey.
We’ll describe the deceleration of the car as a linear function, or the equation of a straight line, and then compute the \(t\)-intercept.
The general form for a linear function is \(y = ax + b\), where \(a\) is the slope of the function and \(b\) is the \(y\)-intercept.
We already know that the gradient of the graph is \(-0.5\), so the function is of the form \[y = -0.5x + b.\]
The graph passes through the point \((70, 15)\), so the function has to satisfy \(15 = -0.5 \times 70 + b\), which yields \(b = 50\).
The function describing the graph for \(t \geq 70\) is therefore given by \[v = -0.5t + 50.\]
The \(t\)-intercept is the solution to \(0 = -0.5t + 50\), which gives \(t = 100\). Therefore, the total time taken for the journey is \(\quantity{100}{s}\).

