Can we find the area inside a parabola, a tangent, and the $x$-axis?

Add to your resource collection Remove from your resource collection Add notes to this resource View your notes for this resource

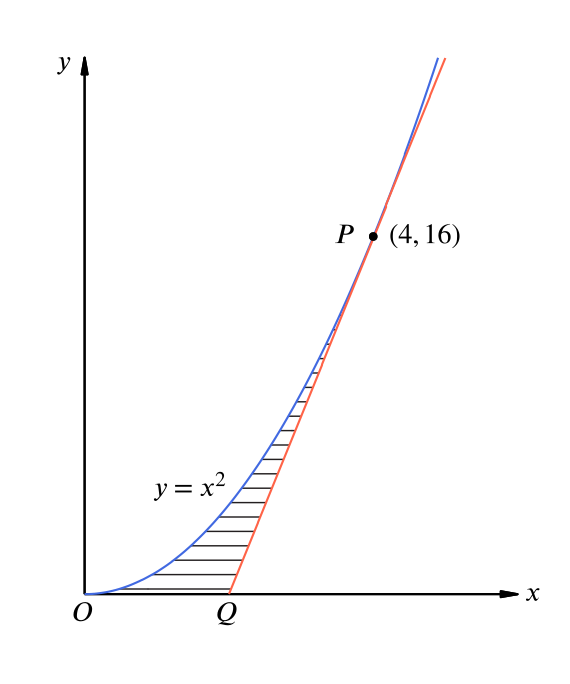
The figure shows part of the curve \(y = x^2\). \(PQ\) is the tangent to this curve at \(P\).

Calculate

1. the coordinates of \(Q\),

The point \(Q\) is the \(x\)-intercept of the tangent. Can we find the equation of the tangent at P, and so find Q?

2. the area of the shaded region.

Could we view this region as the difference of two simpler regions?