Can we find the ratio of the areas of these two sectors?

Add to your resource collection Remove from your resource collection Add notes to this resource View your notes for this resource

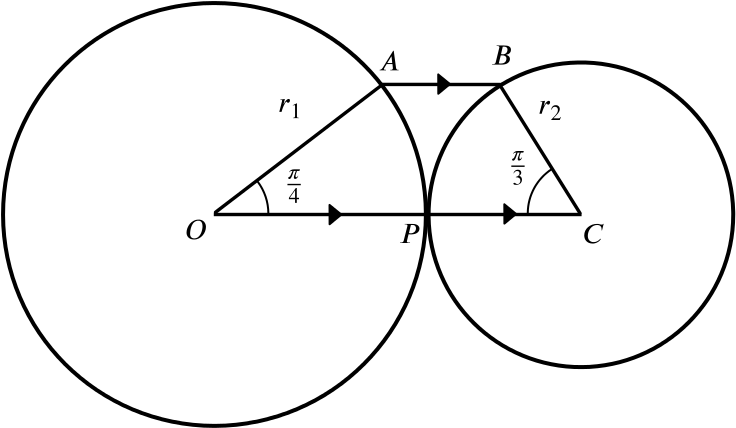
The figure shows two circles, centres \(O\) and \(C\), radii \(r_1\) and \(r_2\), which touch externally at \(P\). Given that \(A\hat{O}P=\dfrac{\pi}{4}\) radians, \(B\hat{C}P = \dfrac{\pi}{3}\) radians and \(AB\) is parallel to \(OPC\), show that \(\dfrac{r_1}{r_2} = \dfrac{\sqrt{6}}{2}\). Hence find the ratio of the areas of the sectors \(OAP\), \(CBP\).