Given that \(a\) and \(b\) are positive and \[4(\log_{10}a)^2+(\log_{10}b)^2=1\] then the greatest possible value of \(a\) is
\(\frac{1}{10}\),
\(1\),
\(\sqrt{10}\),
\(10^{\sqrt{2}}\).
Approach 1
Note first that \(a\) is largest when \(\log_{10}a\) is largest, since \(\log\) is an increasing function. So \(a\) is largest when \((\log_{10}b)^2\) is smallest.
For positive \(b\), the minimum value that \((\log_{10}b)^2\) can take is \(0\) (when \(b=1\)). Then we have \[4(\log_{10}a)^2=1,\] which is equivalent to \[\log_{10}a =\pm \frac{1}{2}.\]
So the largest value this takes is \(\log_{10}a = \frac{1}{2}\), which is when \(a=\sqrt{10}\).
Therefore the answer is (c).
In fact, this also shows that the minimum value for \(\log_{10}a\) happens when \(b = 1\) and \(\log_{10}a = -1/2\). That is, when \(a = 1/\sqrt{10}\).
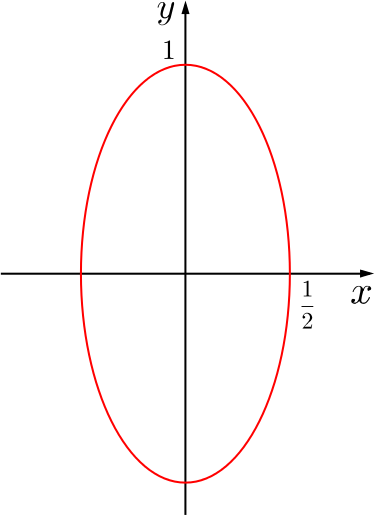
Approach 2
Alternatively, we could take a geometrical approach. Let \(x = \log_{10}a\), \(y = \log_{10}b\). The maximum value for \(x=\log_{10}a\) is achieved when \(a\) takes its maximum value, since \(\log\) is an increasing function.
Our equation now becomes \(4x^2 + y^2 = 1\), which is an ellipse.
From the sketch, we can see that \(x\) has maximum \(\frac{1}{2}\) and so \(a\) has maximum \(\sqrt{10}\), by the same calculation as above.
As before, the answer must be (c).

