Imagine plotting a graph of \(y=2^x\), with \(\quantity{1}{cm}\) to one unit on each axis.
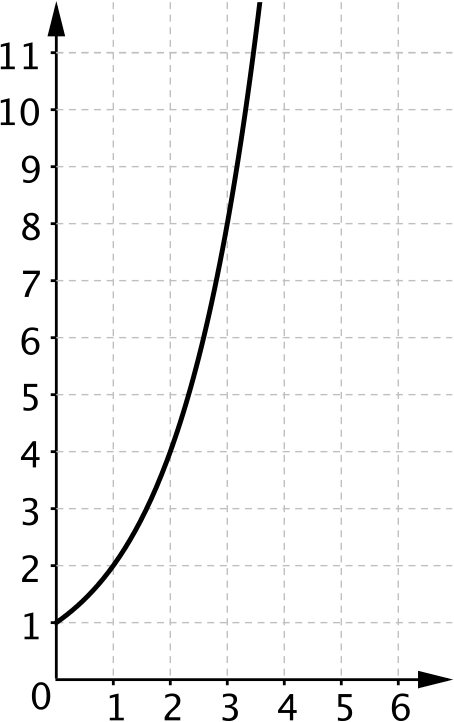
How far along the \(x\)-axis could you go before the graph reached the top of a sheet of paper?
If you extended the graph so the positive \(x\)-axis filled the whole width of a sheet of paper, how tall would the paper have to be?
How far along the \(x\)-axis would you have to go so that the graph was tall enough to reach
- to the top of The Shard in London?
- to the moon?
- to the Andromeda galaxy?
Try to estimate the answers before calculating them and mark them at the appropriate points along a sketch of the \(x\)-axis.
Work out where they should be and then add some other results such as the distances to the sun and other stars. What do you notice?
We have provided a data sheet to work from or you could research suitable data for yourself.

