What is the greatest area of a rectangle inscribed in a given right-angled triangle?
Consider this situation, where \(C\) is a vertex of both the rectangle and the triangle.
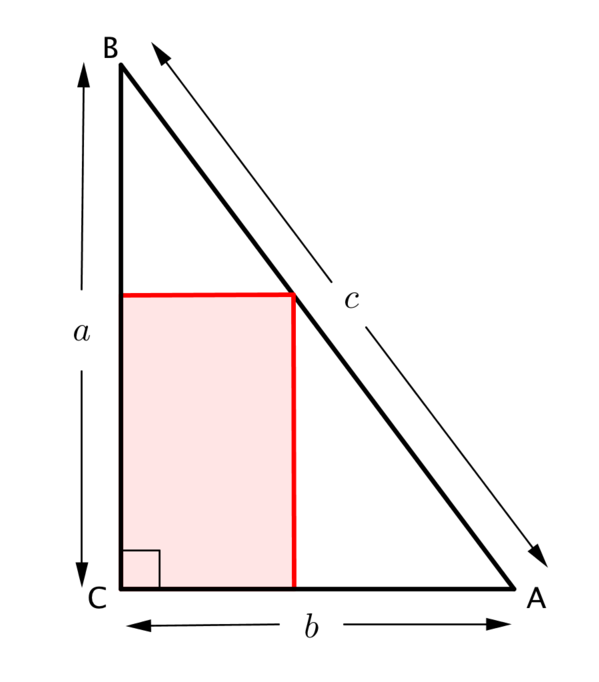
What is the greatest area of a rectangle inscribed in a given right-angled triangle?
Consider this situation, where \(C\) is a vertex of both the rectangle and the triangle.
