Please note that there is an extra printable copy of the student work without comments in case you would like to use this as part of your teaching.
Student work A
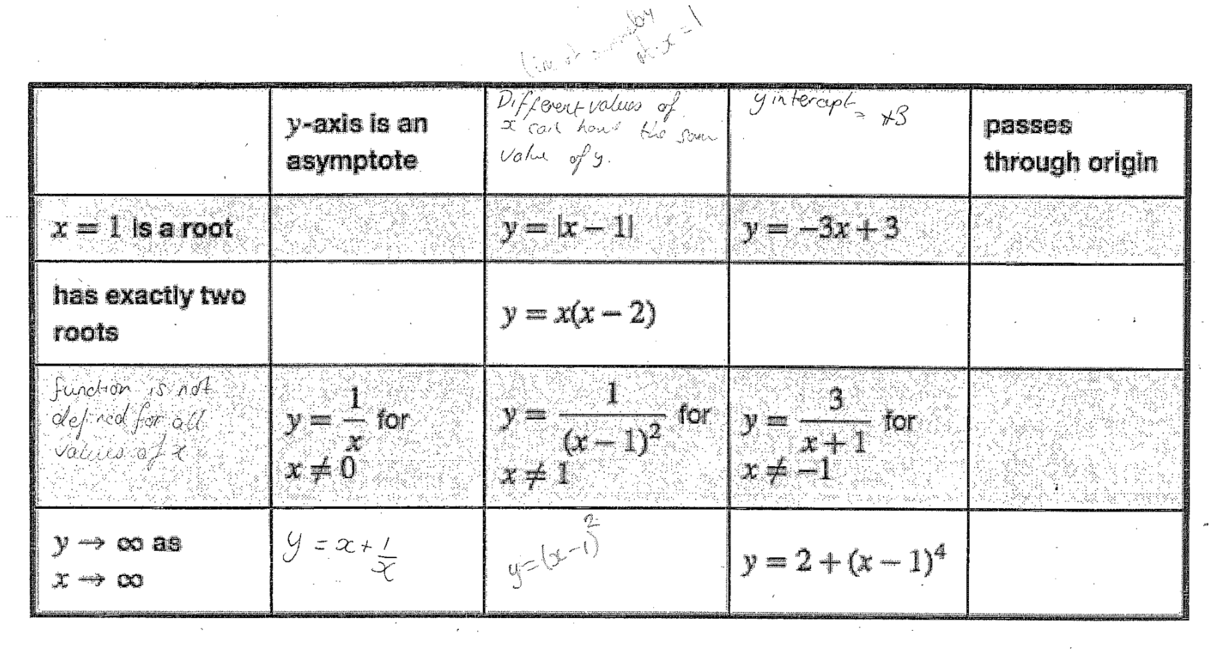
These students focused on identifying missing properties. Some of the properties the students have suggested are in Function squares, which was used as a preliminary task and the two functions that have been entered in the cells are in the set of suggestion cards.
The extra property that has been added to the second column reads “line of symmetry at x=1” and it is not clear whether this was added before or after the function \(y=(x-1)^{2}\) was included.
Student work B
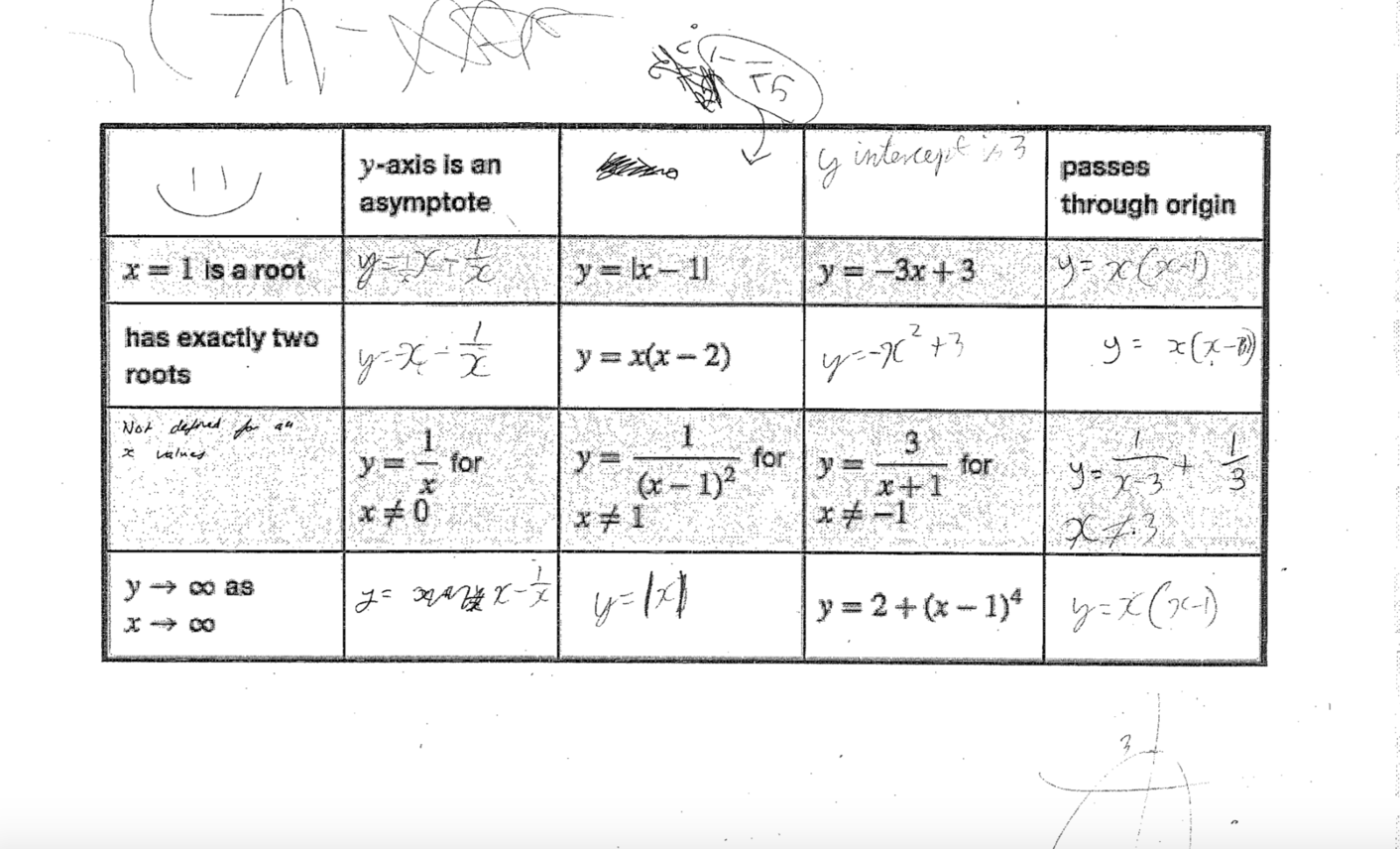
These students have completed the table and then modified some functions in the first and final columns so that they could complete the table with fewer functions (one of the suggested extension tasks). For example, they replaced \(y=x+\dfrac{1}{x}\) with \(y=x-\dfrac{1}{x}\) and replaced \(y=x(x-3)\) with \(y=x(x-1).\) They also sketched graphs to help support their reasoning.
For the second column property the students initially chose \(y\geq 0\) but modified this to \(y\geq -1\) because of the printed function \(y=x(x-2).\)
Student work C
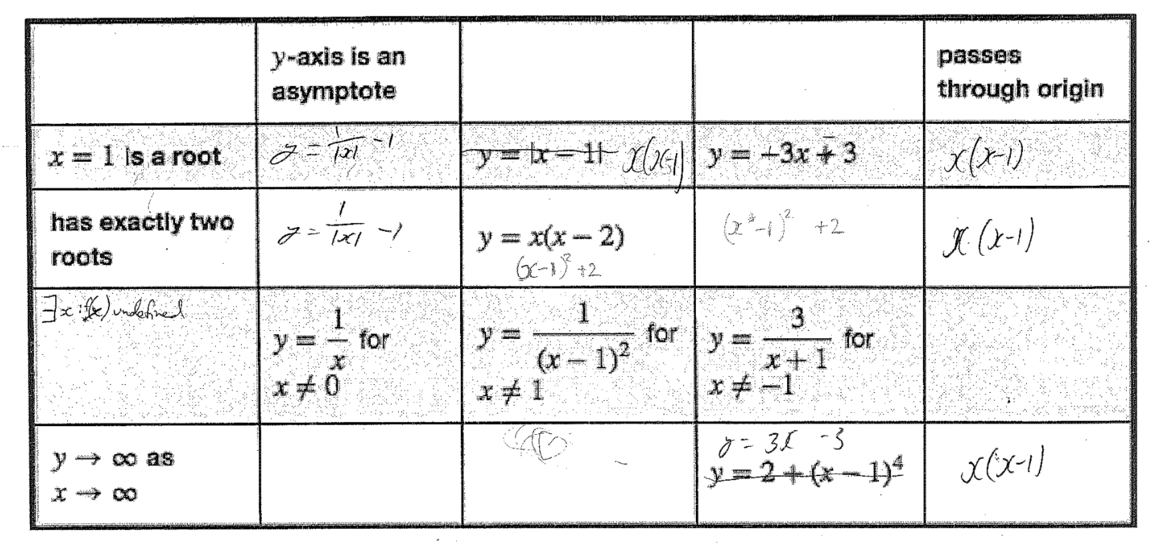
After completing the table once, these students attempted to use as few functions as possible and realized that they could replace some of the printed functions to reduce the total number of functions they needed. The students had used the suggestion cards as part of the preliminary task and it may be worth noting that \(y=\dfrac{1}{\vert x\vert}-1\) and \(y=x(1-x)\) which the students used repeatedly in the extension are transformations of functions on the suggestion cards.
Student work D
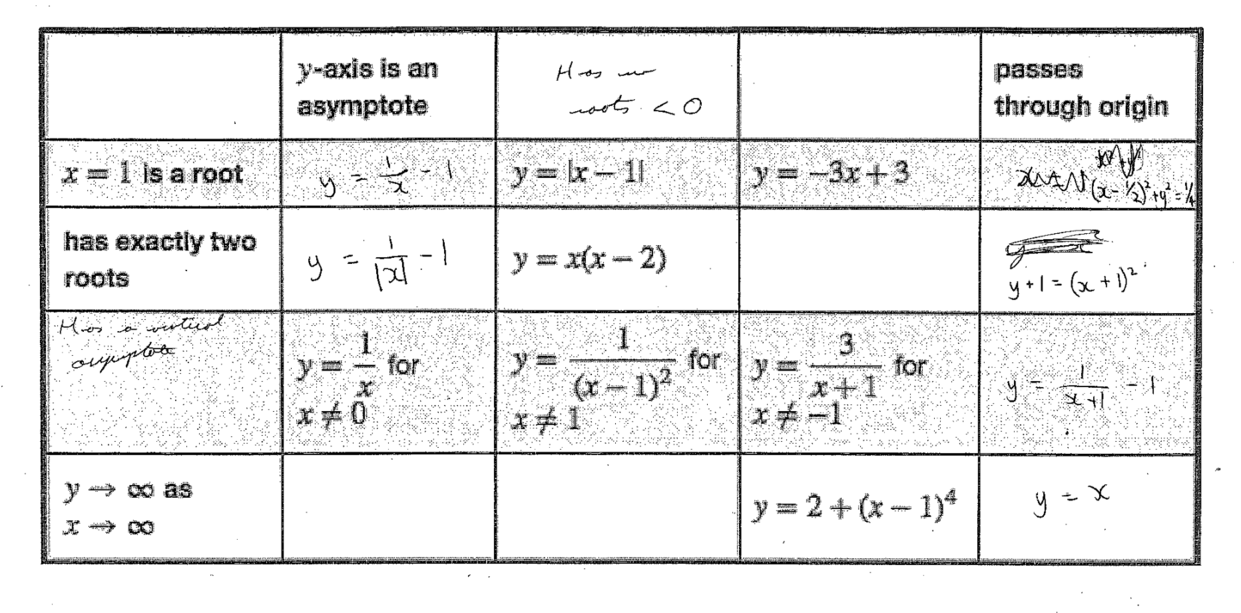
These students have attempted to use different functions in every cell. They also noticed that none of the printed functions in the second column has a negative root. This group suggested the equation \((x-\tfrac{1}{2})^{2}+y^{2}=\tfrac{1}{4}\) which provided the opportunity to discuss what a function is and what its graph must look like. An alternative use for the table would be to ask for the equations of curves rather than restrict the task to functions.
Student work E
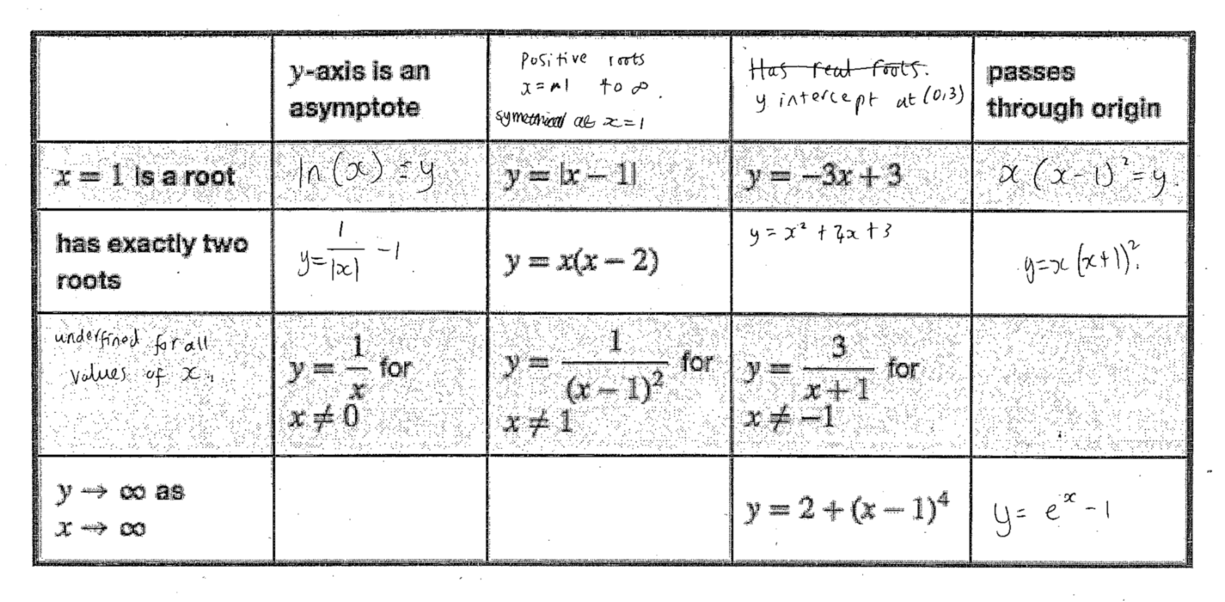
Students in this group drew on their knowledge of exponential and logarithmic functions and also included cubic functions, both of which have repeated roots. Use of graph transformations led to the suggestion of \(y=e^{x}-1\) for a function which tends to infinity as \(x\) tends to infinity, and passes through the origin.
The suggestion “Has real roots” for the third column heading was replaced by “\(y\)-intercept at \((0,3)\)” and this could indicate some confusion between roots and the \(y\)-intercept. The suggested property “positive roots \(x=1\) to \(\infty\)” could also indicate some difficulty with roots, particularly of rational functions.

