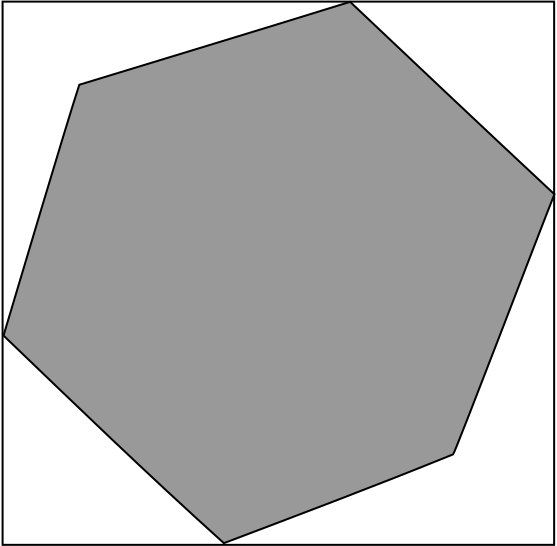
The diagram shows a regular hexagon, with sides of length \(1\), inside a square. Two vertices of the hexagon lie on a diagonal of the square and the other four lie on the edges.
What is the area of the square?
(A) \(\quad 2+\sqrt{3}\qquad\) (B) \(\quad 4\qquad\) (C) \(\quad 3+\sqrt{2}\qquad\) (D) \(\quad 1+\dfrac{3\sqrt{3}}{2}\qquad\) (E) \(\quad\dfrac{7}{2}\)

