Suppose that \(a\), \(b\), \(c\) are integers such that \[a \sqrt{2} + b = c \sqrt{3}.\]
By squaring both sides of the equation, show that \(a = b = c = 0\).
[You may assume that \(\sqrt{2}, \sqrt{3}\) and \(\sqrt{2/3}\) are all irrational numbers. An irrational number is one which cannot be written in the form \(p/q\) where \(p\) and \(q\) are integers.]
Suppose now that \(m\), \(n\), \(M\), \(N\) are integers such that the distance from the point \((m,n)\) to \((\sqrt{2},\sqrt{3})\) equals the distance from \((M,N)\) to \((\sqrt{2},\sqrt{3})\).
Show that \(m=M\) and \(n=N\).
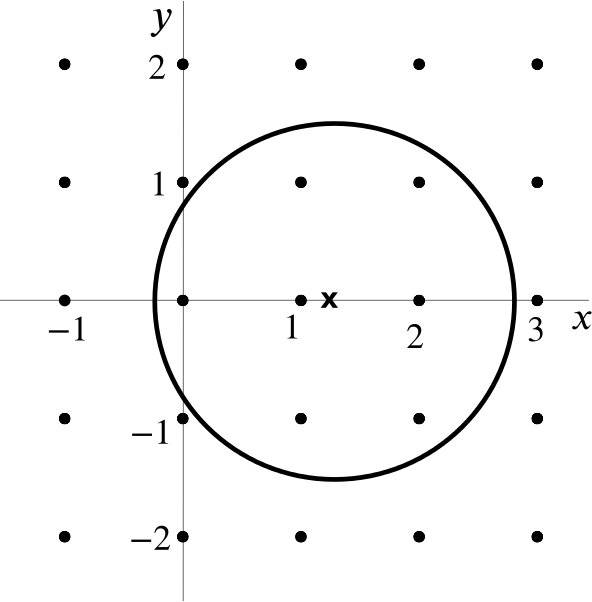
Given real numbers \(a\), \(b\) and a positive number \(r\), let \(N(a,b,r)\) be the number of integer pairs \(x\), \(y\) such that the distance between the points \((x,y)\) and \((a,b)\) is less than or equal to \(r\). For example, we see that \(N(1.2,0,1.5)=7\) in the diagram below.
Explain why \(N(0.5,0.5,r)\) is a multiple of \(4\) for any value of \(r\).
Let \(k\) be any positive integer. Explain why there is a positive number \(r\) such that \(N(\sqrt{2},\sqrt{3},r)=k\).

