\(OABC\) is a square of side \(\quantity{1}{ft.}\). A point \(X\) in \(AB\) and a point \(Y\) in \(BC\) are such that \(AX=\quantity{x}{ft.}\), \(BY=\quantity{kx}{ft.}\) and \(BY\) is longer than \(AX\). For a given value of \(k\), show that the minimum area of the triangle \(OXY\) is \[\quantity{\frac{4k-1}{8k}}{sq.ft.}\]
Don’t get distracted by the choice of feet for units here, the question works perfectly well whatever the units given for length.
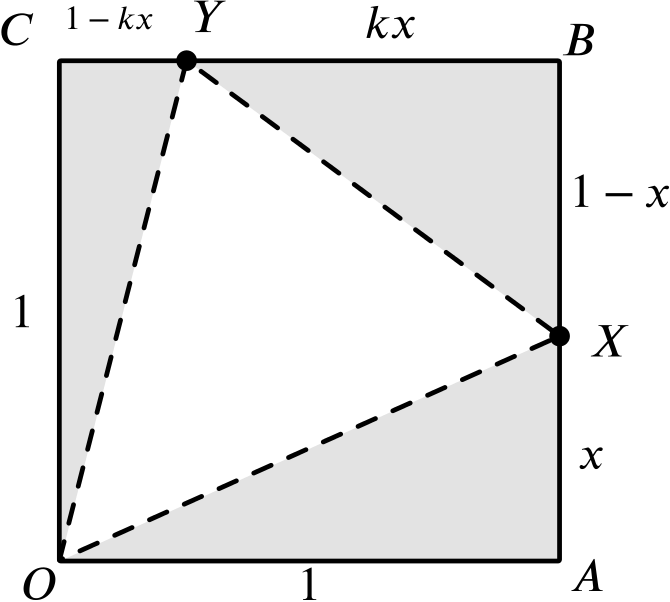
From the picture, we can see that the easiest way to find the area of the triangle \(OXY\) is to subtract the areas of the triangles \(OAX\), \(BXY\) and \(OCY\) from the area of the square \(OABC\).
Writing the area as a function of \(x\), \(A(x)\), we find that \[A(x)=1-\frac{x}{2}-\frac{1}{2}kx(1-x)-\frac{1}{2}(1-kx)=\frac{1}{2}-\frac{x}{2}+\frac{kx^2}{2}.\]
Taking the derivative with respect to \(x\), we find that \[\frac{dA}{dx}=-\frac{1}{2}+kx,\] which is equal to zero when \(x=\dfrac{1}{2k}\). Since \(A(x)\) has a positive \(x^2\) coefficient, this must be a ‘vertex-down’ parabola, and we have a minimum.
Alternatively, since \(A(x)\) is a quadratic, we could have found the minimum point by completing the square.
So we have a minimum value of \[A\left(\dfrac{1}{2k}\right)=\frac{1}{2}-\frac{1}{4k}+\frac{1}{8k}=\frac{1}{2}-\frac{1}{8k}=\frac{4k-1}{8k}.\]
Therefore, for fixed \(k\), the minimum area of the triangle \(OXY\) is \[\quantity{\frac{4k-1}{8k}}{sq.ft.}\]
How does this expression behave as \(k\) varies?

