If $y=f(x)$ has a turning point when $x=\frac{1}{4}$, can we find $\lambda$?

Add to your resource collection Remove from your resource collection Add notes to this resource View your notes for this resource

By expanding \(f(x)\) we obtain \[\begin{align*} f(x) &= x^2-4x+4-\lambda(x^2+3x+2) \\ &= (1-\lambda)x^2-(4+3\lambda)x+4-2\lambda. \end{align*}\] For the equation \(f(x)=0\) to have two equal roots, the discriminant must be zero. So we want to solve \[\begin{align*} 0 &= [-(4+3\lambda)]^2-4(1-\lambda)(4-2\lambda) \\ &= 16+24\lambda+9\lambda^2-4(4-6\lambda+2\lambda^2) \\ &= \lambda^2+48\lambda \\ &= \lambda(\lambda+48), \end{align*}\]

and so \(\lambda = 0\) or \(\lambda=-48\).

When \(\lambda=2\), we have \(f(x)=-x^2-10x\).

We find the maximum of \(f(x)\) by completing the square.

We can write \(f(x)=-(x+5)^2+25\), and since \(-(x+5)^2\) is strictly negative, we see that the maximum value of \(f(x)\) is \(25\), which occurs when \(x=-5\).

We have \[f'(x)=2(1-\lambda)x-(4+3\lambda).\]

We know that \(f'\left(\dfrac{1}{4}\right)=0\), since there is a turning point at \(x=\dfrac{1}{4}\).

So \[2(1-\lambda)\left(\frac{1}{4}\right)-4-3\lambda=0,\] and so \[1-\lambda-8-6\lambda=0,\] on multiplying the equation by \(2\). This simplifies to \(\lambda = -1\).

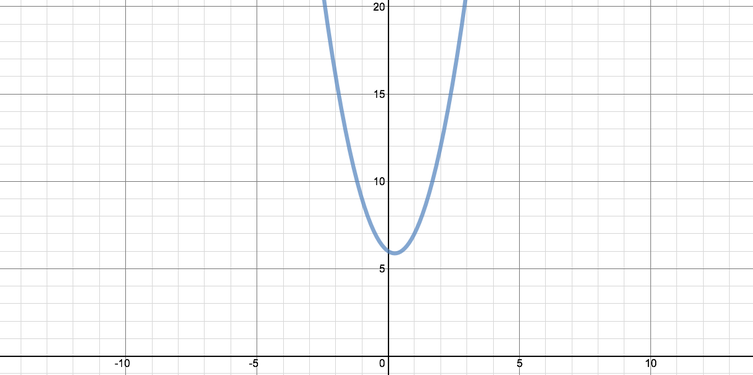
For this value of \(\lambda\), we have \(f(x)=2x^2-x+6\).

To sketch this graph we observe that the \(y\)-intercept is \(6\), and that \(x=\dfrac{1}{4}\) gives a minimum, since the coefficient of the \(x^2\) term is positive.

We calculate that \[f\left(\frac{1}{4}\right)=\frac{2}{16}-\frac{1}{4}+6=\frac{2-4+96}{16}=\frac{94}{16}=\frac{47}{8}.\]