In the diagram below \(f(x)\) is a linear function.
The area of the green shaded region (above the \(x\)-axis) is \(12\) square units.
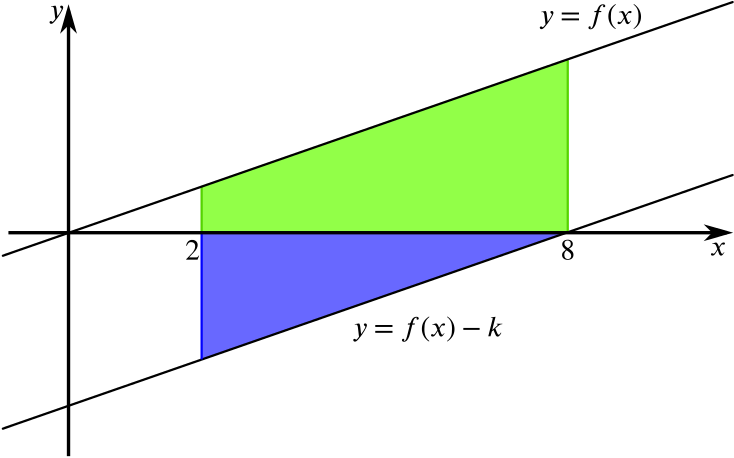
What is the area of the blue shaded region (below the \(x\)-axis)?
Take a step back, look at the image and write down anything that you know about it (without doing any calculations).
\(f(x)\) passes through the origin.
\(f(x)\) is a straight line.
\(f(x)\) could be written in the form \(ax + b\) (where \(b=0\) in this case).
\(y=f(x)-k\) is parallel to \(y=f(x)\) (it is a vertical translation by \(k\) units downwards).
The green region is a trapezium of ‘height’ \(6\) units.
The blue region is a triangle of ‘base’ or ‘height’ \(6\) units.
The combination of the green and blue shaded regions produces a parallelogram.
The blue triangle could sit ‘on top of’ the green trapezium to create a rectangle.
The area of the blue triangle must be less than \(12\) square units.


