We usually think of using the trapezium rule when we know what the function is or have a scale on the axes, but what can you say when you don’t have this information?
For each of the graphs below, what would happen if you used the trapezium rule to estimate the area between the curve and the \(x\)-axis?
You could start by trying to decide for each graph whether the trapezium rule will give you an underestimate or an overestimate. The graphs are available to print out and you may find it helpful to draw on them. You could also cut them up and sort them if you wish.
There are many things to notice about these graphs and how the area between the curve and the \(x\)-axis could be approximated using the trapezium rule. We’ve suggested trying to decide about under or over estimates because thinking about this may spark other ideas or questions. Keep a note of these so that you can investigate them further.
Graph 1 
Graph 2 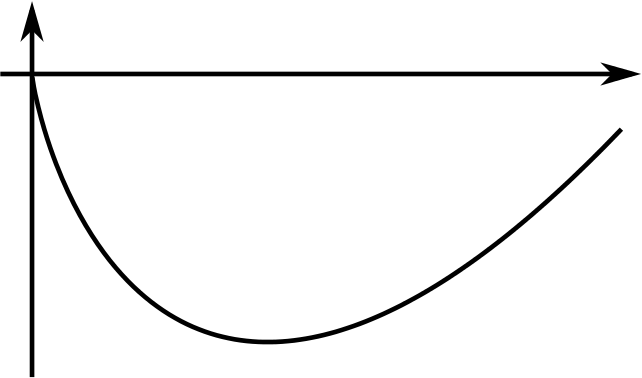
Graph 3 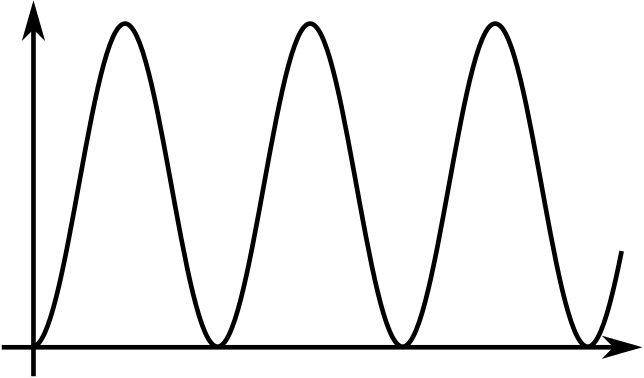
Graph 4 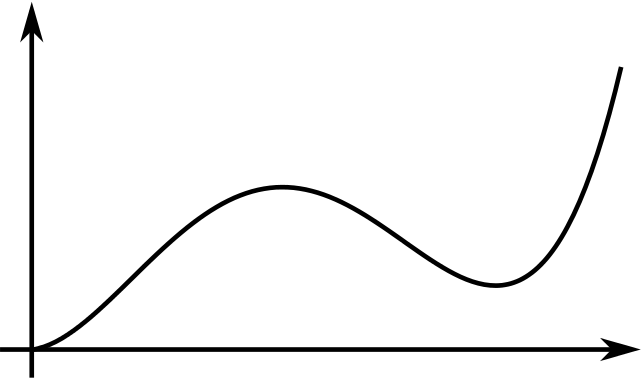
Graph 5 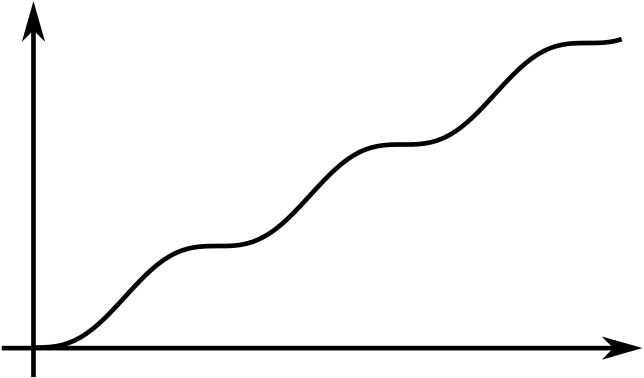
Graph 6 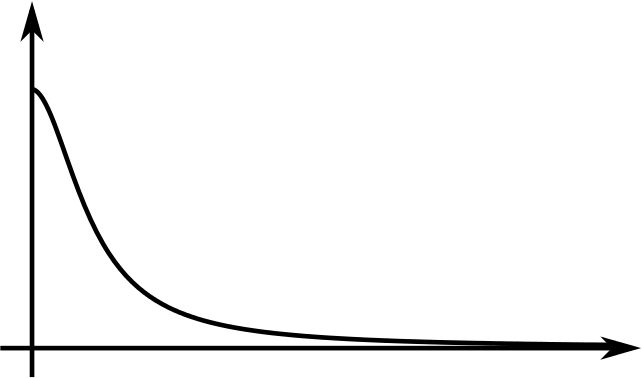
Graph 7 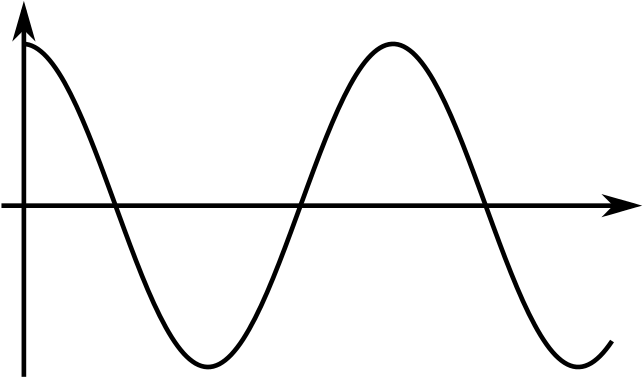
Graph 8 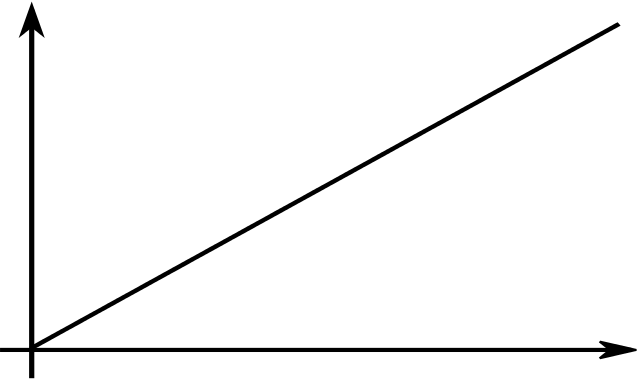
Graph 9 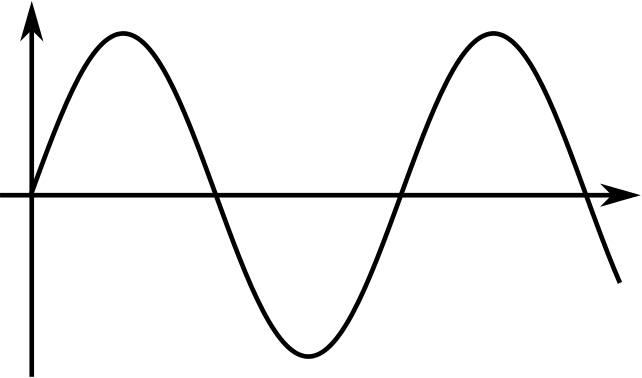
Graph 10 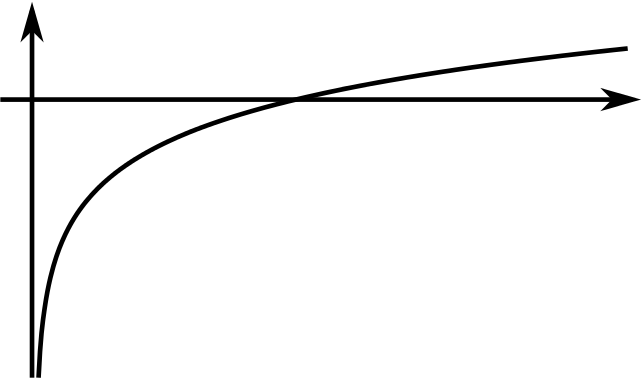
Graph 11 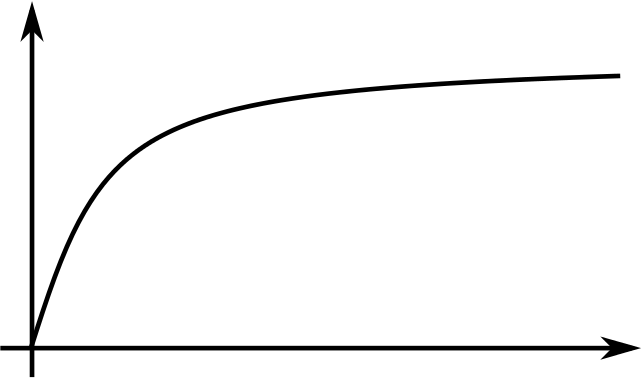
Graph 12 
What other ideas did you have?
What questions could you try to answer next?
Please note that these graphs are also used in Gradient match.

