For the functions given below, try to decide whether or not the function is odd or even. If the function is neither odd nor even, is its graph symmetric in some other way?
We have provided the functions as cards for you to cut out and sort.
\(f(x)=2x+1\)
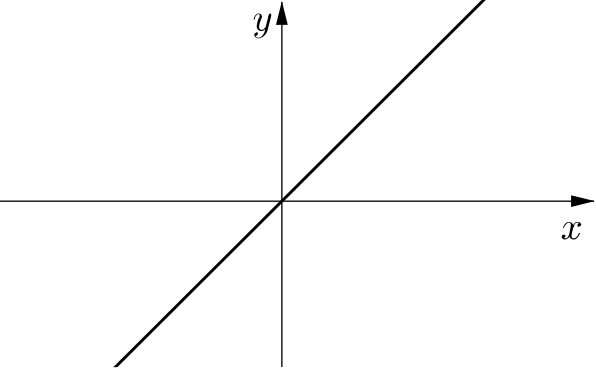\(f(x)=x\)
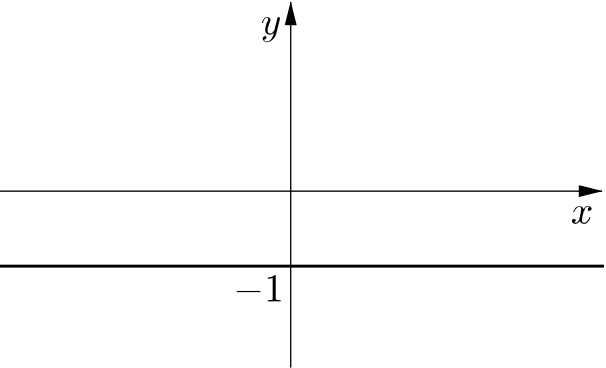\(f(x)=-1\)
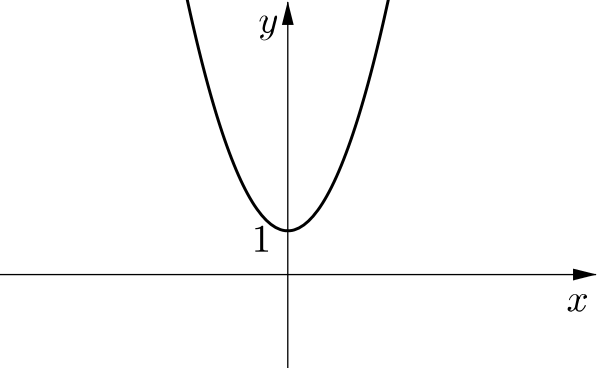\(f(x)=x^2+1\)
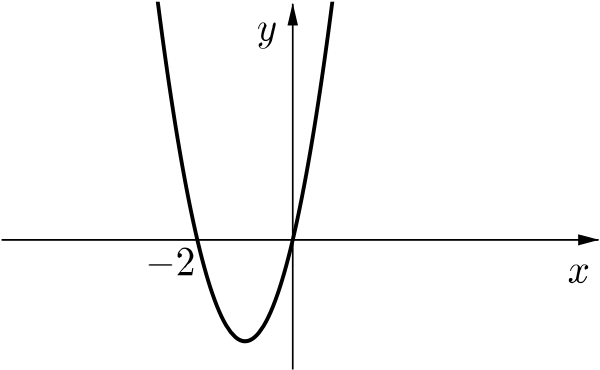\(f(x)=2x^2+4x\)
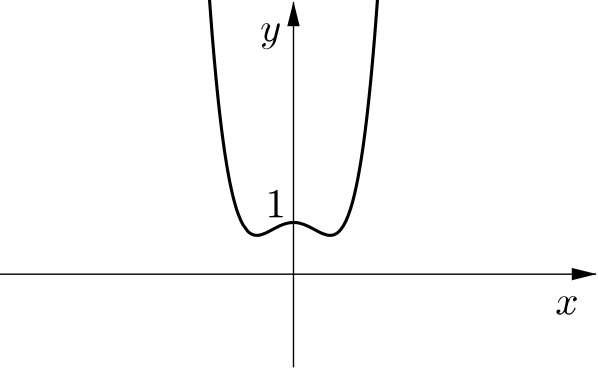\(f(x)=1-x^2+x^4\)
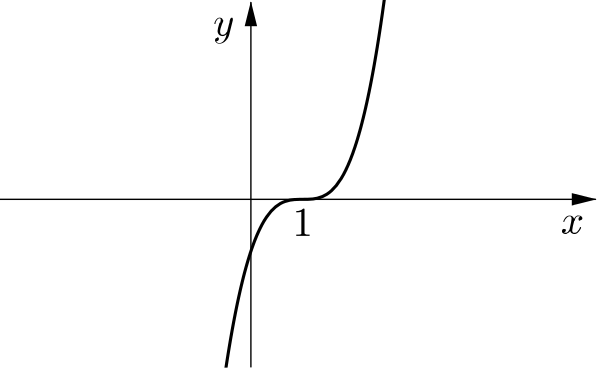\(f(x)=(x-1)^3\)
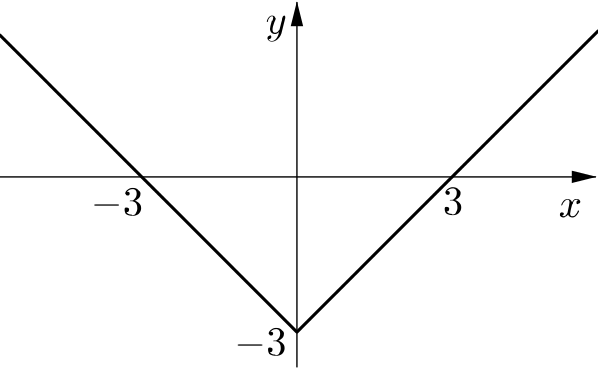\(f(x)=\vert x \vert-3\)
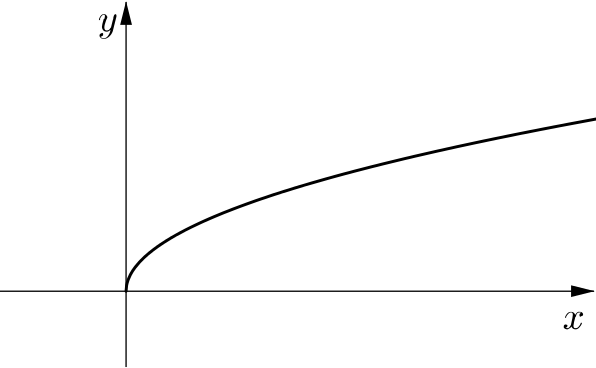\(f(x)=\sqrt{x}\)
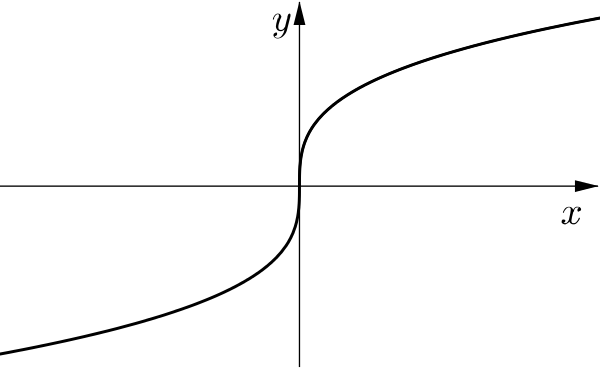\(f(x)=\sqrt[3]{x}\)
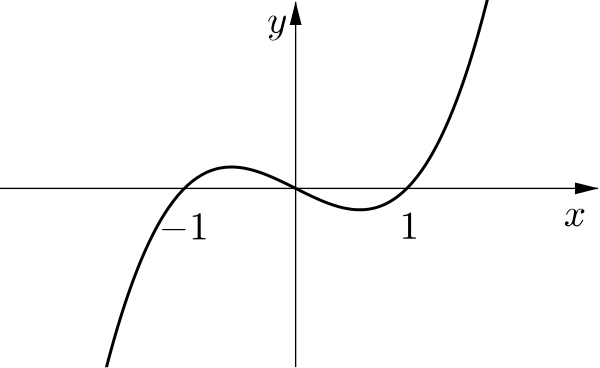\(f(x)=x^3-x\)
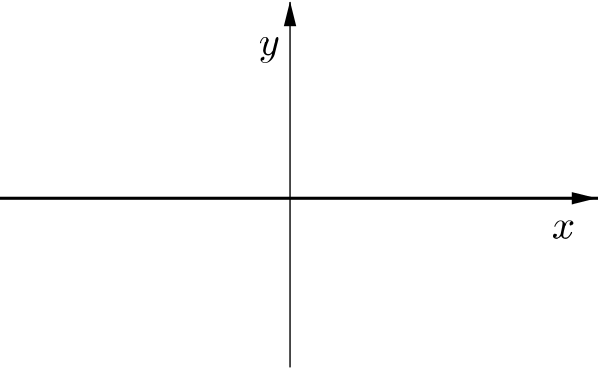\(f(x)=0\)
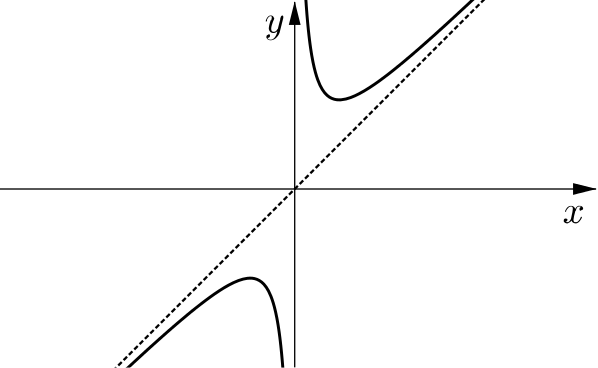\(f(x)=x+\dfrac{1}{x}\)
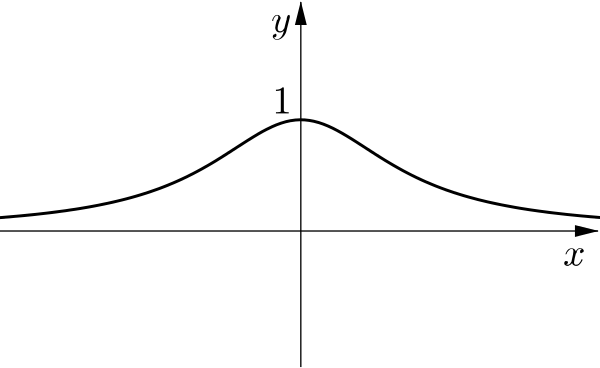\(f(x)=\dfrac{1}{x^2+1}\)
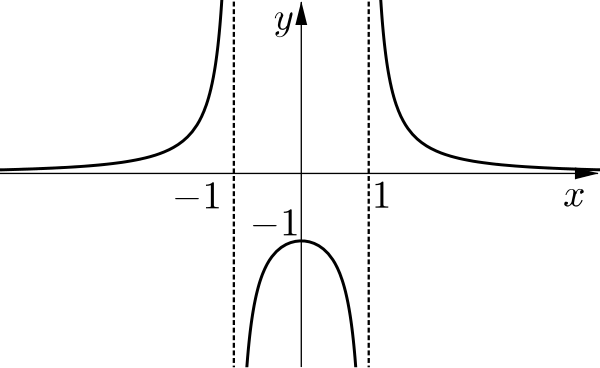\(f(x)=\dfrac{1}{x^2-1}\)
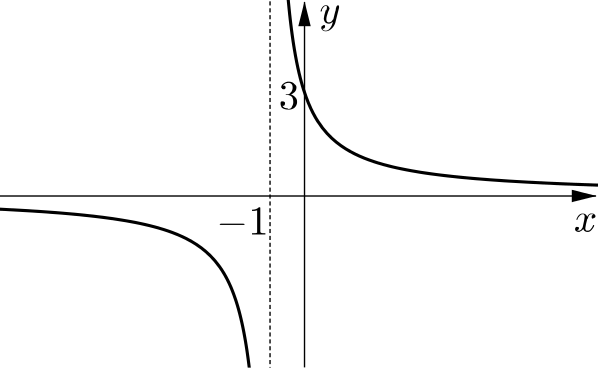\(f(x)=\dfrac{3}{1+x}\)
