- In the following figure, two semicircles have been drawn, one on the side \(AB\) of the triangle, and the other on the side \(AC\) of the triangle (with centre \(O\)). What is the area of the blue (shaded) lune which is bounded by the two semicircles?

Not having any idea how to calculate the area of a lune, the best we can do to get started is to calculate the areas we can.
First of all, we will colour some of the other regions to make it easier to talk about them:
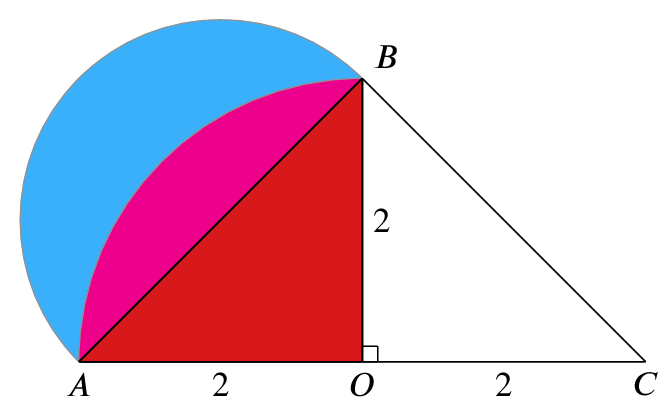
The red area is a right-angled triangle with area \(\frac{1}{2}\times2\times2=2\).
The red and magenta regions together make a quarter of a circle of radius \(2\), so have combined area \(\frac{1}{4}\pi\times 2^2=\pi\).
The blue and magenta regions together make a semicircle whose diameter is the hypotenuse of the triangle. This hypotenuse has length \(\sqrt{2^2+2^2}=\sqrt{8}=2\sqrt{2}\), and so the radius of the semicircle is \(\sqrt{2}\). Therefore the blue and magenta regions have a combined area \(\frac{1}{2}\pi(\sqrt{2})^2=\pi\).
Putting these together, we find \[\text{red}+\text{magenta}=\text{blue}+\text{magenta}\;({}=\pi)\] so that the red and blue areas are equal.
Thus the blue lune has area \(2\), the same as the red triangle.
As a bonus, can you construct a square on the diagram with the same area as the blue lune, using only a straight edge (ruler) and compasses? This is called the quadrature (making into a square) of the lune.
The square must have area \(2\), so side length \(\sqrt{2}\). One way to construct this is to note that the hypotenuse of the red triangle has length \(2\sqrt{2}\), so half of it has length \(\sqrt{2}\). We can find the midpoint of \(AB\) using the standard straight edge and compasses construction (see, for example, BBC Bitesize if you don’t remember how to do this). Then joining this point to \(O\) gives two sides of the square. Repeating this on the side \(BC\) completes the job, as shown by the red square in this diagram:
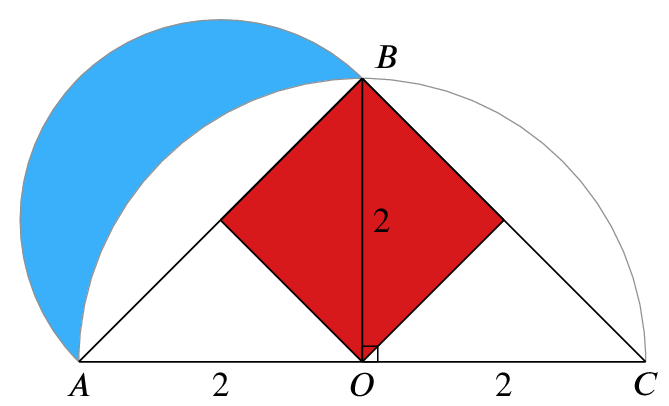
Another way to think about this is that the red triangle is a quarter of the square \(ABCD\), where \(D\) is a fourth point opposite \(B\). Then the red triangle has the same area as a square with half the edge length of \(ABCD\).
Note that the actual side lengths are mostly unimportant to this question: the blue lune will always have the same area as the red triangle in this configuration.
- In the following figure, three semicircles have been drawn, one on each of the sides of the right-angled \(6\)-\(8\)-\(10\) triangle. What is the total area of the two coloured (shaded) lunes in the drawing?

We’ll colour the various regions as we did before.

The red triangle has area \(\frac{1}{2}\times 6\times 8=24\).
The two magenta regions and the red region together form a semicircle with radius \(5\), so area \(\frac{1}{2}\pi\times 5^2=\frac{25}{2}\pi\).
The blue and left magenta region form a semicircle with radius \(3\), so area \(\frac{1}{2}\pi\times 3^2=\frac{9}{2}\pi\).
The green and right magenta region form a semicircle with radius \(4\), so area \(\frac{1}{2}\pi\times 4^2=\frac{16}{2}\pi\).
Adding these together as before gives \[\text{red}+\text{both magentas}= \text{blue}+\text{green}+\text{both magentas}\;({}=\frac{25}{2}\pi)\]
Therefore the blue and green lunes together equal the red triangle, which has area \(24\).
For some historical background on this problem, and a note about question 3, see the Historical background section.

