Exit fullscreen mode

Thinking about Geometry

Review question

Can we use similar triangles to find this side?
Add to your resource collection Remove from your resource collection Add notes to this resource View your notes for this resource

Ref: R5081

Printable/supporting materials Printable version Fullscreen mode Teacher notes

Question

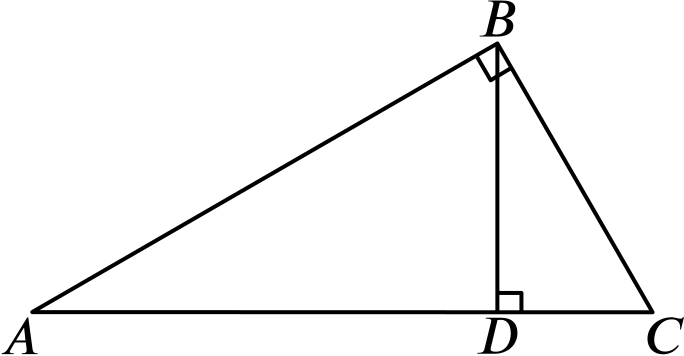
A picture of the triangle A B C, where A B C is a right-angle and B C is the hypotenuse of another triangle B C D where d lies on A C

In the diagram, \(\widehat{ABC}=\widehat{BDC}=90^\circ\).

Write down an angle equal to \(\widehat{CBD}\).
Given that \(AC=\quantity{10}{cm}\) and \(BC=\quantity{7}{cm}\), use similar triangles to calculate \(CD\).