Exit fullscreen mode

Thinking about Geometry

Review question

Can we find the areas within the semicircles?
Add to your resource collection Remove from your resource collection Add notes to this resource View your notes for this resource

Ref: R6699

Printable/supporting materials Printable version Fullscreen mode Teacher notes

Question

State the theorem of Pythagoras concerning the three sides of a right-angled triangle.

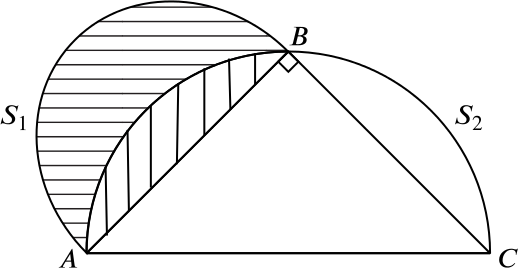
A diagram showing the triangle $ABC$ and the semicircles $S_1$ and $S_2$.

The diagram shows an isosceles triangle \(ABC\) right-angled at \(B\). Semicircles \(S_1\) and \(S_2\) are drawn on \(AB\) and \(AC\) as diameter, as shown.

Explain why \(S_2\) passes through \(B\).
If \(AB = \quantity{2x}{cm}\), show that the area shaded vertically is \(\quantity{\tfrac{1}{2}(\pi - 2)x^2}{cm^2}\).
Prove that the area shaded horizontally is half the area of the triangle \(ABC\).