The sum of the interior angles of a polygon with \(n\) sides is \((2n-4)\) right angles. Deduce that each interior angle of a regular polygon is \(\left( 2 - \dfrac{4}{n} \right)\) right angles.
Three regular polygons, two having \(n\) sides and one having \(p\) sides, fit together exactly at a common vertex, as shown:
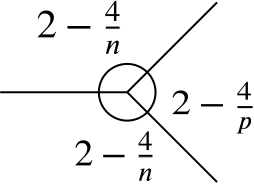
By using the fact that the sum of the three angles shown is four right angles, prove that \[ \frac{4}{n} + \frac{2}{p} = 1. \]
Show that this formula can be rearranged into the form \[ p = \frac{2n}{n-4}. \] By inserting into this formula various values of \(n\), list all the pairs of positive integers \((n,p)\) for which \(n < 12\) which satisfy this equation. By means of a sketch, interpret one of these solutions where \(n\) and \(p\) are different in terms of three regular polygons meeting at a common vertex.

