The graphs of \(y = x^3 - x\) and \(y = m(x-a)\) are drawn on the axes below. Here \(m>0\) and \(a \le -1\).
The line \(y=m(x-a)\) meets the \(x\)-axis at \(A = (a,0)\), touches the cubic \(y=x^3 -x\) at \(B\) and intersects again with the cubic at \(C\). The \(x\)-coordinates of \(B\) and \(C\) are respectively \(b\) and \(c\).
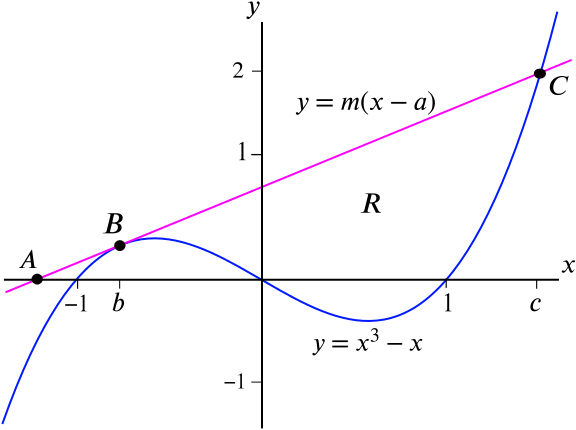
- Use the fact that the line and cubic touch when \(x=b\), to show that \(m=3b^2-1\).
What do we know about curves and lines which touch at some point? How can that help us to answer this question?
- Show further that \[a = \frac{2b^3}{3b^2 - 1}.\]
Can we find \(m\) simply in terms of \(a\) and \(b\)? Could we drop a vertical from \(B\)?
- If \(a=-10^6\), what is the approximate value of \(b\)?
Can you sketch a graph to show this situation?
- Using the fact that \[x^3 - x - m(x-a) = (x-b)^2 (x-c)\] (which you need not prove), show that \(c=-2b\).
Why is this true? For which values of x is this true? So this tells us that …
- \(R\) is the finite region bounded above by the line \(y=m(x-a)\) and bounded below by the cubic \(y = x^3 - x\). For what value of \(a\) is the area of \(R\) largest?
Show that the largest possible area of \(R\) is \(\dfrac{27}{4}\).
How does the area of \(R\) change as \(a\) changes?
Or… can we find a formula for the area? When we’ve done so, how can we maximise this?

