Trigonometry: Triangles to Functions
Building blocks

Going round in circles
Add to your resource collection Remove from your resource collection Add notes to this resource View your notes for this resource

Printable/supporting materials Printable version Fullscreen mode Teacher notes

Triangles

Here are three triangles with some side lengths and angles marked. Express the missing side lengths in terms of \(\sin \theta\), \(\cos \theta\) and \(\tan \theta.\)

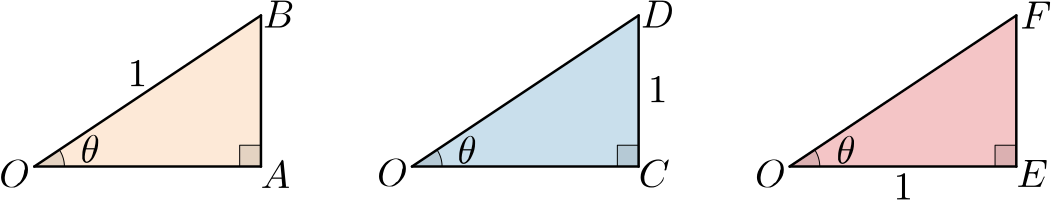
three right-angled triangles with angle theta marked and one side of length 1.

  • How can using side length ratios for \(\sin \theta\), \(\cos \theta\) and \(\tan \theta\) help you?

  • These triangles are all similar. How can you use this to help you?

What do you notice if you compare the results of the two approaches?

Functions such as \(\dfrac{1}{\cos \theta}\) are often called reciprocal trig functions and have special names

\[\dfrac{1}{\cos \theta}=\sec \theta \quad \quad \dfrac{1}{\sin \theta}=\cosec \theta \quad \quad \dfrac{1}{\tan \theta}=\cot \theta.\]

These are short for “secant”, “cosecant” and “cotangent” respectively; they are usually referred to by their short names.

We now have six trigonometric functions. From the side lengths in the triangles above, what relationships can you find between these functions?

Previous Next
Last updated 06-Mar-17
Add to your collection Add the current resource to your resource collection
Email Twitter
Terms Cookies Privacy
Rich resources for teaching A level mathematics
Copyright © and Database Right 2013-2024 University of Cambridge
All rights reserved
University of Cambridge logo
Department for Education logo